Once upon a time, I found myself totally stuck in a really tough spot. I was a super young widow with these two little troublemakers…
Female Fans More Intelligent Than Males
It’s official: female sports fans have a higher average IQ score than their male counterparts. This has been a heated debate amongst sports fans, but…
Slot Games
Slot Games at Planet 7 Casino Who doesn’t love slot games and the huge payouts they offer? But what if you prefer not to visit…
Bingo Games
Bingo is known as one of the most extraordinary social games that involves plenty of interaction and chatter with other players. There are various sorts…
Fulltilt Poker
Full Tilt Poker is widely recognized as one of the largest and most reputable online poker websites in the world. Unfortunately, in 2011, the website…
Las Vegas USA Casino Review
It is not surprising that Las Vegas is a major gambling hub, however, the saying “What happens in Vegas” no longer stays in Vegas; it…
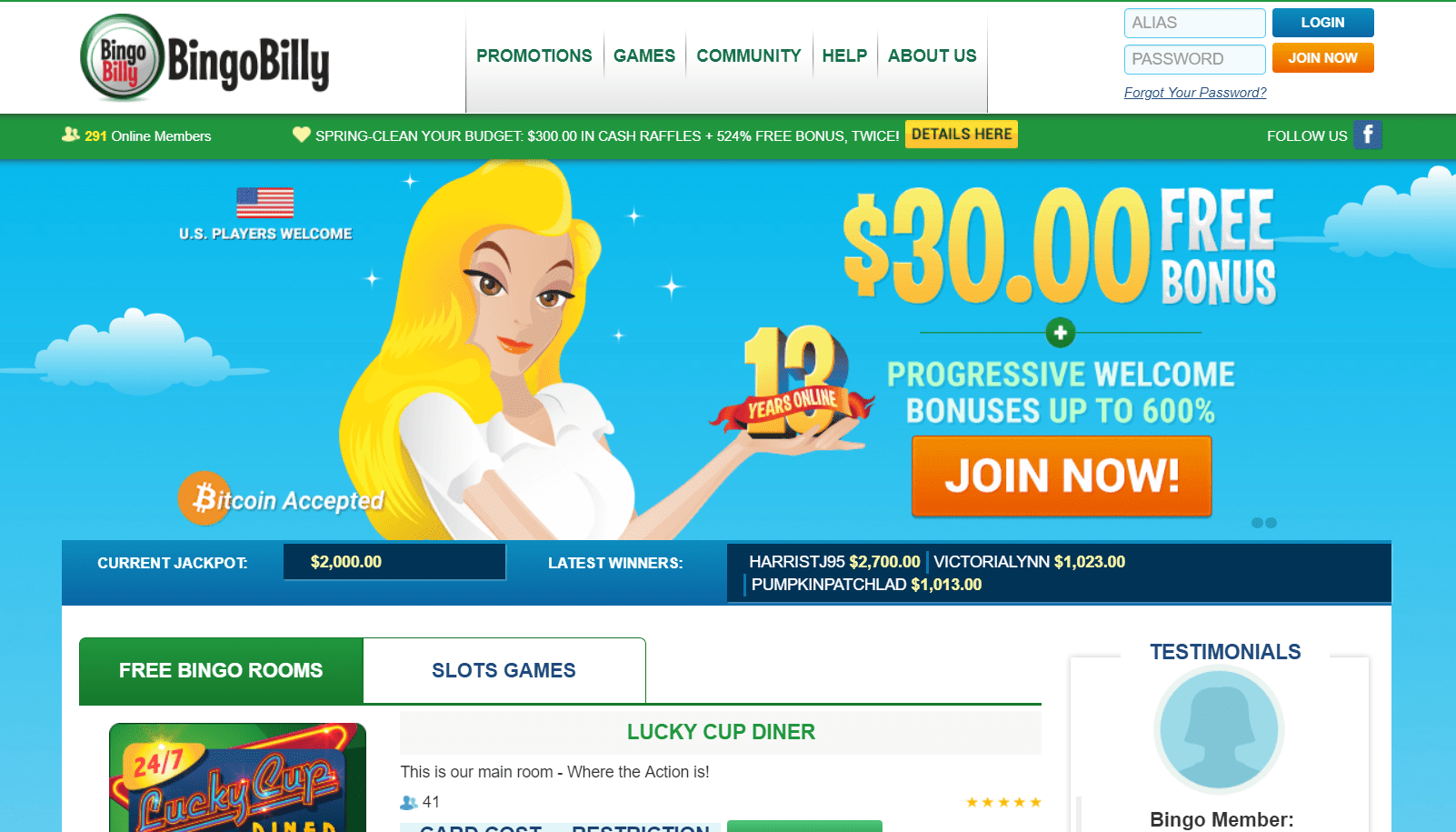
Bingo Billy Casino Review
Bingo Billy Casino offers approximately 100 games, primarily from Betsoft. Unfortunately, they do not offer any live games and only have 1 table game available….
Sloto Cash Casino Review
Sloto’Cash is one of the internet’s most well-known online casinos. In the fall of 2011, Sloto’Cash was re-launched as an RTG casino. Thus, it now…
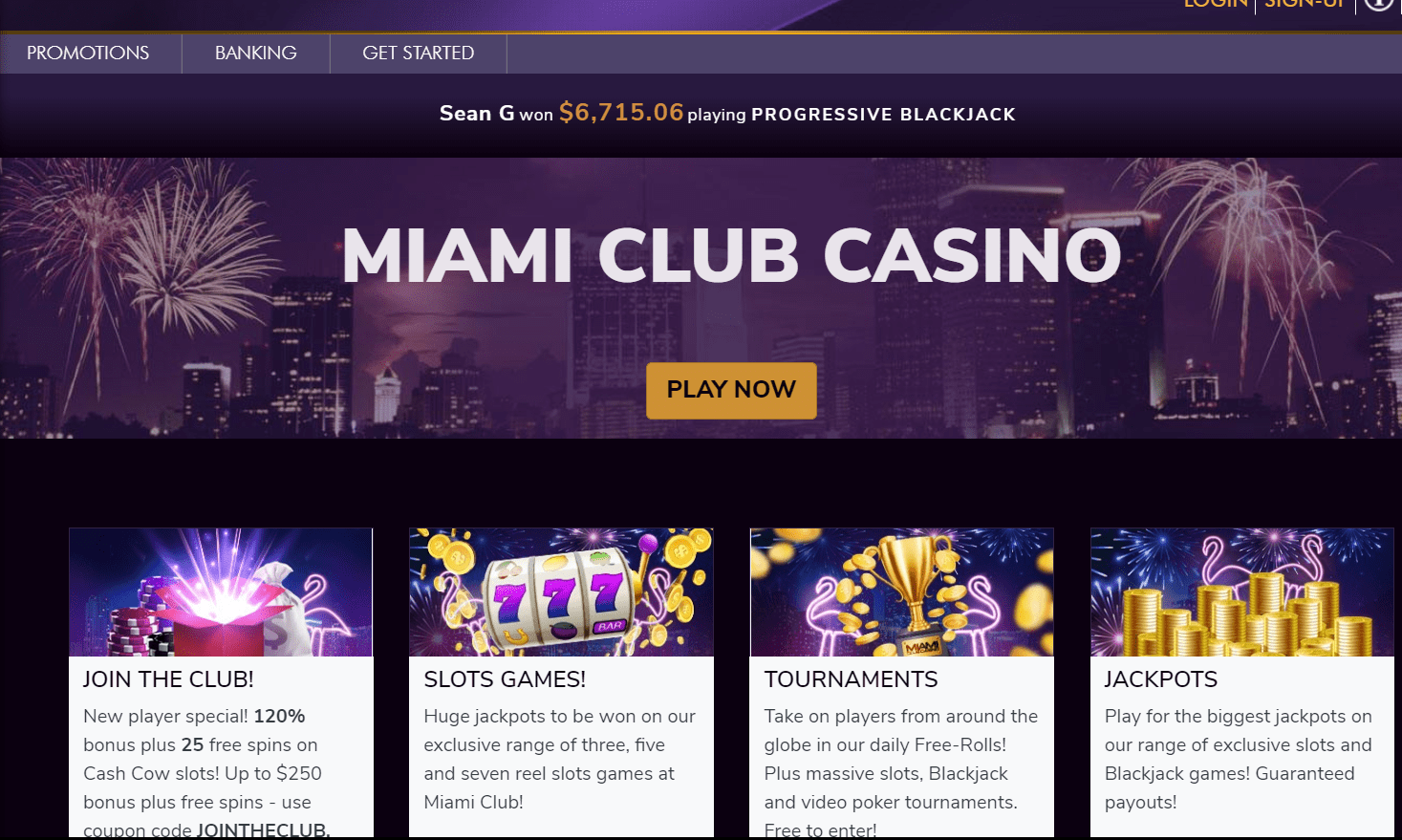
Miami Club Casino Review
Miami Club Casino is a recently established online casino that was launched in 2012. It is operated by Deckmedia N.V., a well-known operator in the…
Black Diamond Casino Review
Black Diamond Casino is a renowned online casino that was launched by the Deckmedia group in 2008. It became a sensation in the internet gaming…